
The characteristic polynomial of an n × n complex matrix factors into n linear factors, and so the algebraic multiplicies of all eigenvalues for any n × n complex matrix sum to n. ■Įigenvalues, eigenvectors, and diagonalizable matrices are defined for complex matrices in the same manner as for real matrices. If W, Z are n × n complex matrices, then | W Z | = | W | | Z |, | W T | = | W |, and | W ∗ | = | W ¯ | = | W | ¯. ■Īn n × n complex matrix W is nonsingular iff | W| ≠ 0 iff rank( W) = n. The rank and determinant of complex matrices are computed in the same manner as for real matrices. The Gaussian elimination method and Gauss-Jordan Method apply to systems of complex linear equations. If A has nonreal eigenvalues, then A is not diagonalizable when considered as a real matrix, but A may be diagonalizable when considered as a complex matrix. Let A be a complex matrix with all entries real. ■Ī complex n × n matrix A is not diagonalizable if the number of fundamental eigenvectors obtained in the Diagonalization Method for A does not equal n. The Diagonalization Method applies to complex matrices. ■Ī complex matrix A is diagonalizable if and only if there is a nonsingular complex matrix P such that P −1 AP = D is a diagonal matrix. That is, the sum of the algebraic multiplicities of the eigenvalues of A equals n. If A is an n × n complex matrix, the eigenvalues of A are the complex roots of the characteristic polynomial p A ( x ) = x I n − A of A, which factors over the complex numbers into n linear factors.

Such a nonzero complex vector v is an eigenvector for A associated with λ.
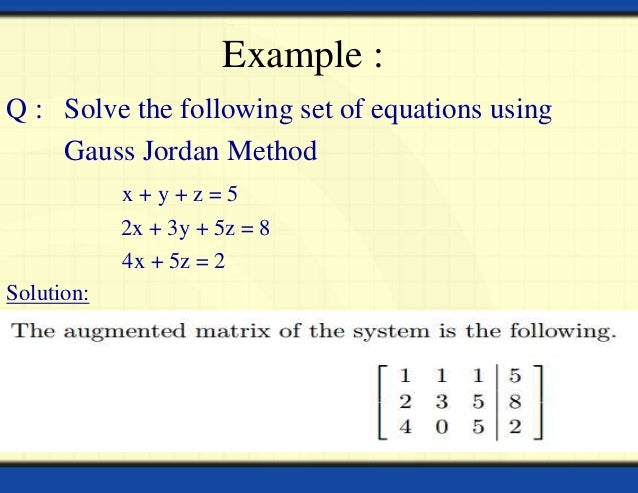
If A is an n × n complex matrix, then λ ∈ C is an eigenvalue for A if and only if there is a nonzero vector v ∈ C n such that Av = λ v. If W, Z are n × n complex matrices, then | WZ| = | W|| Z|, | W T| = | W|, and | W * | = | W ¯ | = | W | ¯. ■Īn n × n complex matrix W is nonsingular iff | W|≠0 iff rank( W) = n. ■įor a complex matrix, its rank, row space, inverse (if it exists) and determinant can all be computed using the same techniques valid for real matrices. Gaussian Elimination and the Gauss-Jordan Method can be used to solve systems of complex linear equations.


 0 kommentar(er)
0 kommentar(er)
